Hi all. I’m Xenocho also known as Remmystarr from Eden. The reason this post is made was due to streams of questions in the forum and Discord regarding calculations. As I am quite experienced in calculations on stats, I thought I would write this mini guide to elucidate and perhaps provide an understanding to questions involving gear calculation and also balancing of stats on jades.
Terminology clarification
- The first thing I must clarify is the terminology of gear. I will be mentioning ‘gear’ a lot in this write-up and hence I should make it clear:
- Gears are items which are part of the equipment worn by the character, which is shown in the character info tab (default key: P); this includes armors, weapons, accessories, origins, all cash equipment including engravings, mount, heraldries and talismans.
- This explanation is the same when it comes to stats as well.
Understanding
1. What is the ATK shown in the stat window? 
2. Effective attack
3. Law of diminishing return
A layman term which I used to explain this is as follows:
Terminology clarification
- The first thing I must clarify is the terminology of gear. I will be mentioning ‘gear’ a lot in this write-up and hence I should make it clear:
- Gears are items which are part of the equipment worn by the character, which is shown in the character info tab (default key: P); this includes armors, weapons, accessories, origins, all cash equipment including engravings, mount, heraldries and talismans.
- This explanation is the same when it comes to stats as well.
Understanding
1. What is the ATK shown in the stat window?
Using this example, there are 3 parts when you mouse-over the attack section (this also applies to the stats info window):

- White number: attack derived from the main stat of the character
In terms of calculation, the white stat value is simply obtained by looking at the total stats and calculating. For the engineer, AGI is the main stat and the conversion is 0.75 ATK per AGI.
This gives: 12,676 * 0.75 = 9,507
In addition, STR is a sub stat and the conversion is 0.25 ATK per STR.
This gives: 4,819 * 0.25 = 1204.75
In total, 9,507 + 1,204 = 10,711 ATK
This gives: 12,676 * 0.75 = 9,507
In addition, STR is a sub stat and the conversion is 0.25 ATK per STR.
This gives: 4,819 * 0.25 = 1204.75
In total, 9,507 + 1,204 = 10,711 ATK
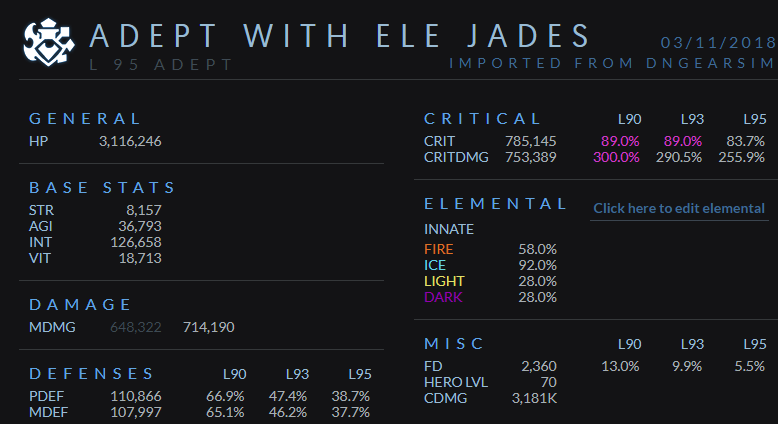
- Green number: attack contributed by gear
When we describe the ATK contributed by gear in the green value, they are actually a total of the ATK values, as well as the % increment as well. The example is as shown below:
Let’s call the total ATK from gear as A and the % values as B.
A = Neris Cannon + Threaded Loop + Jade + Heraldry + Cash Equipment + Hero Level
A = 32,728 + 2,187 + 519 + 12,896 + 100 + 4340
A = 52,770
B = Jade + Title + Cash Equipment
B = 4% + 6% + 1.7%
B = 11.7%
Green value = A + (A + White)*B
Green value = 52,770 + (52,770 + 10,711)*11.7%
Green value = 52,770+ 7,427.277
Green value = 60,197 ATK
Let’s call the total ATK from gear as A and the % values as B.
A = Neris Cannon + Threaded Loop + Jade + Heraldry + Cash Equipment + Hero Level
A = 32,728 + 2,187 + 519 + 12,896 + 100 + 4340
A = 52,770
B = Jade + Title + Cash Equipment
B = 4% + 6% + 1.7%
B = 11.7%
Green value = A + (A + White)*B
Green value = 52,770 + (52,770 + 10,711)*11.7%
Green value = 52,770+ 7,427.277
Green value = 60,197 ATK
- Blue number: attack contributed by skill ATK %
The blue value is actually the calculation of damage from skill based ATK % increments. These include Rock of Blessing (10%), Class Masteries (varies), Breaking Point (10%) and so forth. These increments are calculated based on the green and white values, which are both contributed by gear.
In our example, the character is a gear master. Hence, its class mastery is 5% ATK increment of the green and white values. Hence:
Blue value = (White value + Green value) * ATK %
Blue value = (10,711 + 60,197) * 5%
Blue value = 70,908 * 5%
Blue value = 3,545.4 ATK
Because this calculation is multiplied using the final values of the gear ATK, we do not include it in our gear ATK calculations. In a way, the ATK % can also be viewed as a form of multiplier.
Also, take note that this blue value has a limit of 100% of gear. This means that class with high ATK % from passives and class masteries need to be aware that further ATK % buffs will have high diminishing returns or even hitting the 100% cap. Refer to the picture below for evidence (credits to myon for highlighting this again).

In our example, the character is a gear master. Hence, its class mastery is 5% ATK increment of the green and white values. Hence:
Blue value = (White value + Green value) * ATK %
Blue value = (10,711 + 60,197) * 5%
Blue value = 70,908 * 5%
Blue value = 3,545.4 ATK
Because this calculation is multiplied using the final values of the gear ATK, we do not include it in our gear ATK calculations. In a way, the ATK % can also be viewed as a form of multiplier.
Also, take note that this blue value has a limit of 100% of gear. This means that class with high ATK % from passives and class masteries need to be aware that further ATK % buffs will have high diminishing returns or even hitting the 100% cap. Refer to the picture below for evidence (credits to myon for highlighting this again).

2. Effective attack
a. As you know, there are many other parameters or stats which you can push. These are Critical, Critical Damage, Elemental Attack and Final Damage.
b. Due to the nature of calculation, where these parameters act as multipliers, they are calculated separately and the order of which is calculated first does not matter. Also, 1 = 100%, 0.1 = 10% in calculations; they are the same thing.
- The full formula is as shown:
- Hence, the E-ATK of a character with 100,000 ATK with 10% FD and 5% Ele is calculated as follows:
- Understanding how these calculations work is important for understanding damage optimization in later sections.
c. CDMG calculation is slightly different, due to the nature of CDMG heavily reliant on critical chance. As a result, having a low critical chance significantly reduces the impact of critical damage.
- These examples are as shown below using the formula [CDMG * CritChance + (1 – CritChance%)]:
- Hence, as you can see, although Remmy had cap crit damage, the actual multiplier ends up to be very close to Aethriel’s basic crit damage. This was due to the influence of critical, which plays a huge role.
d. As we can calculate the E-ATK of different setup and gears, we can also calculate to identify which has a better output.
- This difference can be expressed in terms of percentage (%) as shown below:
- Hence, Remmy’s E-ATK is 20% higher than Aethriel’s.
Critical is the chance of dealing double damage or higher, which is increased by raising the AGI or the critical stat directly. Without the influence of critical damage, critical hits deal 2x of non-critical hits. Critical is capped at 89%.
Critical damage is a stat which further increases the damage output of critical hits by up to 100%. This results in the maximum damage of a critical hit to deal 3x of non-critical hits. Critical damage is increased either by STR or INT stats, or by raising the critical damage stat directly. Unlike other calculations, critical damage has no 1 + CDMG formula due to its value shown in the stat window already being formatted for calculation.
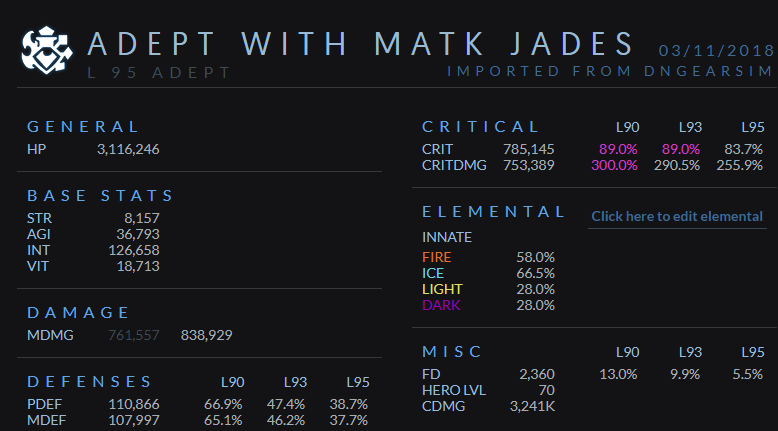
Elemental attack is a stat which increases your damage output for that respective element. Currently, due to the availability of ECJs, any class can deal elemental damage and hence, this stat becomes useful for damage boosting. Although elemental attack is derived from both gears and skills, they are added together during calculations. Currently, there is no limit for elemental attack for both gears and skills.
Final damage is a stat which increases your damage output. This stat is highly valued due to being a different multiplier which can be used to boost damage output. For each cap, there is a certain amount of FD which a character can stack before they hit a limit of 100%. This limit is specifically for gear FD. FD can also be sourced from skills such as Erratic Power or skill heraldries, such as damage plates, which add 20% FD for that particular skill. Ultimately, the total FD is added up and can exceed 100%.
Critical damage is a stat which further increases the damage output of critical hits by up to 100%. This results in the maximum damage of a critical hit to deal 3x of non-critical hits. Critical damage is increased either by STR or INT stats, or by raising the critical damage stat directly. Unlike other calculations, critical damage has no 1 + CDMG formula due to its value shown in the stat window already being formatted for calculation.
Elemental attack is a stat which increases your damage output for that respective element. Currently, due to the availability of ECJs, any class can deal elemental damage and hence, this stat becomes useful for damage boosting. Although elemental attack is derived from both gears and skills, they are added together during calculations. Currently, there is no limit for elemental attack for both gears and skills.
Final damage is a stat which increases your damage output. This stat is highly valued due to being a different multiplier which can be used to boost damage output. For each cap, there is a certain amount of FD which a character can stack before they hit a limit of 100%. This limit is specifically for gear FD. FD can also be sourced from skills such as Erratic Power or skill heraldries, such as damage plates, which add 20% FD for that particular skill. Ultimately, the total FD is added up and can exceed 100%.
b. Due to the nature of calculation, where these parameters act as multipliers, they are calculated separately and the order of which is calculated first does not matter. Also, 1 = 100%, 0.1 = 10% in calculations; they are the same thing.
- The full formula is as shown:
ATK * (1 + FD) * (1 + Ele %) * [CDMG * CritChance + (1 – CritChance%)]
E-ATK = 100,000 * (1 + 0.1) * (1 + 0.05)
E-ATK = 100,000 * 1.1 * 1.05
E-ATK = 115,500
c. CDMG calculation is slightly different, due to the nature of CDMG heavily reliant on critical chance. As a result, having a low critical chance significantly reduces the impact of critical damage.
- These examples are as shown below using the formula [CDMG * CritChance + (1 – CritChance%)]:
Remmy has a CDMG of 300% and CritChance of 50%, while Aethriel has a CDMG of 210% and a CritChance of 89%.
Remmy E-ATK = 3*0.5 + (1-0.5)
Remmy E-ATK = 1.5 + 0.5
Remmy E-ATK = 2.0
Aethriel E-ATK = 2.1 * 0.89 + (1 - 0.89)
Aethriel E-ATK = 1.869 + 0.11
Aethriel E-ATK = 1.979
d. As we can calculate the E-ATK of different setup and gears, we can also calculate to identify which has a better output.
- This difference can be expressed in terms of percentage (%) as shown below:
Remmy’s E-ATK is 240,000, while Aethriel’s E-ATK is 200,000.
Percentage difference = (Remmy’s E-ATK / Aethriel’s E-ATK) - 1
Percentage difference = (240,000 / 200,000) -1
Percentage difference = 1.2 – 1
Percentage difference = 0.2 or 20%
3. Law of diminishing return
a. What is it?
Based on Wikipedia, diminishing returns is stated as the decrease in the marginal (incremental) output of a production process as the amount of a single factor of production is incrementally increased, while the amounts of all other factors of production stay constant.
A layman term which I used to explain this is as follows:
In analogy:
Case 1: you have 2 apples, you get 1 apple.
Case 2: you have 10 apples, you get 1 apple.
In case 1, you get 1 apple to make 3 apples. 3 apples versus 2 apples is a 50% increment from adding 1 apple.
In case 2, you get 1 apple to make 11 apples. 11 apples versus 10 apples is only a 10% increment from adding 1 apple.
In both cases, you add the same amount of apples but the "gain" in case 1 is higher because you have less apples in the first place.
Case 1: you have 2 apples, you get 1 apple.
Case 2: you have 10 apples, you get 1 apple.
In case 1, you get 1 apple to make 3 apples. 3 apples versus 2 apples is a 50% increment from adding 1 apple.
In case 2, you get 1 apple to make 11 apples. 11 apples versus 10 apples is only a 10% increment from adding 1 apple.
In both cases, you add the same amount of apples but the "gain" in case 1 is higher because you have less apples in the first place.
.
b. How does this apply to DN?
- In DN, the same can be said when adding stats onto already high stats. For example, when you add 20% FD onto a character with 100% FD, the calculation is as follows:
- In DN, the same can be said when adding stats onto already high stats. For example, when you add 20% FD onto a character with 100% FD, the calculation is as follows:
FD Increment = [New FD / Old FD] - 1
FD Increment = [(20 + 200)/ (200)] - 1
FD Increment = 10%, which is half of the initial 20% added.
FD Increment = [(20 + 200)/ (200)] - 1
FD Increment = 10%, which is half of the initial 20% added.
- In comparison, adding 20% FD onto a character with 10% FD, the calculation is as follows:
FD Increment = [New FD / Old FD] - 1
FD Increment = [(20 + 110)/(110)] -1
FD Increment = 18%, which is relatively close to the initial 20% added.
FD Increment = [(20 + 110)/(110)] -1
FD Increment = 18%, which is relatively close to the initial 20% added.
- While the 100% FD example shows high diminishing returns, it is not true to say that diminishing returns occurs only at cap FD values. In fact, not obtaining the full 20% is already a sign that diminishing returns is already present. Hence, diminishing returns always occurs in calculations. The effect simply becomes more obvious with higher stat values.







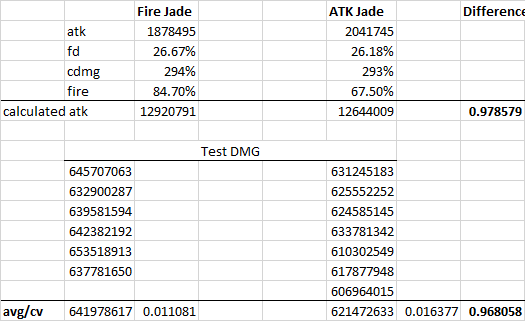
 Thank you and have a good day.
Thank you and have a good day.
Comment